Question 1

Algebra (Grade 5, Easy)

Help

Solve x + 2 = 6

x = 3

x = 4

x = 6

x = 8

Don't waste this chance to learn

Question 2

Algebra (Grade 5, Easy)

Help

Solve x + 5 = 11

x = 16

x = 10

x = 8

x = 6

Use pen and paper to work out the answer

Introduction to Algebra

Algebra is great fun - you get to solve puzzles!

A Puzzle

What is the missing number?

−

OK, the answer is 6, right? Because 6 − 2 = 4. Easy stuff.

Well, in Algebra we don't use blank boxes, we use a letter (usually an x or y, but any letter is fine). So we write:

−

It is really that simple. The letter (in this case an x) just means "we don't know this yet", and is often called the unknown or the variable.

And when we solve it we write:

Why Use a Letter?

	Because:
	it is easier to write "x" than drawing empty boxes (and easier to say "x" than "the empty box").
	if there are several empty boxes (several "unknowns") we can use a different letter for each one.

So x is simply better than having an empty box. We aren't trying to make words with it!

And it doesn't have to be x, it could be y or w ... or any letter or symbol we like.

How to Solve

Algebra is just like a puzzle where we start with something like "x − 2 = 4" and we want to end up with something like "x = 6".

But instead of saying "obviously x=6", use this neat step-by-step approach:

Work out what to remove to get "x = ..."
Remove it by doing the opposite (adding is the opposite of subtracting)
Do that to both sides

Here is an example:

We want to remove the "-2"	To remove it, do the opposite, in this case add 2:	Do it to both sides:	Which is ...	*Solved!*

Why did we add 2 to both sides?

To "keep the balance"...

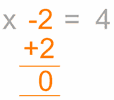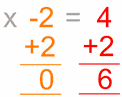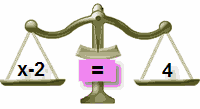In Balance

Add 2 to Left Side

Out of Balance!

Add 2 to Right Side Also

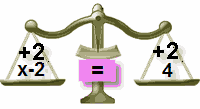In Balance Again

Just remember this:

To keep the balance, what we do to one side of the "="
we should also do to the other side!

See this in action at the Algebra Balance Animation.

Another Puzzle

Solve this one:

Start with:	x + 5 = 12

What we are aiming for is an answer like "x = ...", and the plus 5 is in the way of that! We can cancel out the plus 5 by doing a subtract 5 (because 5−5=0)

So, let us have a go at subtracting 5 from both sides:	x+5 −5 = 12 −5

A little arithmetic (5−5 = 0 and 12−5 = 7) becomes:	x+0 = 7

Which is just:	x = 7
	*Solved!*
(Quick Check: 7+5=12)

Have a Try Yourself

Algebra

Algebra is great fun - you get to solve puzzles!

With computer games you play by running, jumping or finding secret things. Well, with Algebra you play with letters, numbers and symbols, and you also get to find secret things!

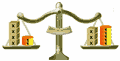
And once you learn some of the "tricks", it becomes a fun challenge to work out how to use your skills in solving each "puzzle".