Add caption

Scaling

We can use ratios to scale drawings up or down (by multiplying or dividing).

The height to width ratio of the Indian Flag is 2:3 So for every 2 (inches, meters, whatever) of height there should be 3 of width.
If we made the flag 20 inches high, it should be 30 inches wide. If we made the flag 40 cm high, it should be 60 cm wide (which is still in the ratio 2:3)

Example:
To draw a horse at 1/10th normal size, multiply all sizes by 1/10th

This horse in real life is 1500mm high and 2000 mm long, so the ratio of its height to length is

1500 : 2000

What is that ratio when we draw it at 1/10th normal size?

Answer: 1500 : 2000 = 1500×1/10 : 2000×1/10 = 150 : 200

We can make any reduction/enlargement we want that way.

Big Foot?

Allie measured her foot and it was 21cm long, and then she measured her Mother's foot, and it was 24cm long.

"I must have big feet, my foot is nearly as long as my Mom's!"

But then she thought to measure heights, and found she is 133cm tall, and her Mom is 152cm tall.

In a table this is:

	Allie	Mom
Length of Foot:	21cm	24cm
Height:	133cm	152cm

The "foot-to-height" ratio in fraction style is:

Allie:

21 133

Mom:

24 152

So the ratio for Allie is 21 : 133

By dividing both values by 7 we get 21/7 : 133/7 = 3 : 19

And the ratio for Mom is 24 : 152

By dividing both values by 8 we get 24/8 : 152/8 = 3 : 19

The simplified "foot-to-height" ratios are now:

Allie:

3 19

Mom:

3 19

"Oh!" she said, "the Ratios are the same".

"So my foot is only as big as it should be for my height, and is not really too big."

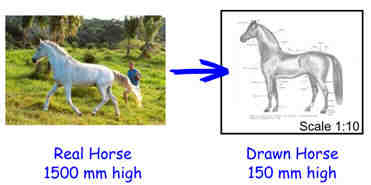
A drawing that shows a real object with accurate sizes reduced or enlarged by a certain amount (called the scale).

The scale is shown as the length in the drawing, then a colon (":"), then the matching length on the real thing.

Example: this drawing has a scale of "1:10", so anything drawn with the size of "1" would have a size of "10" in the real world, so a measurement of 150mm on the drawing would be 1500mm on the real horse.

Drag the butterfly to change its scale

Scale   1 : 1

© 2015 MathsIsFun.com v0.8