Lines and Angles

Point

A point is an exact location. It has no size, only position.

Drag the points below (they are shown as dots so you can see them, but a point really has no size at all!)

Points usually have a name, often a letter like "A", or even "W"

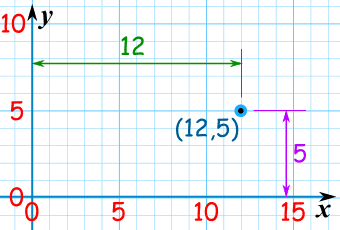
The exact location of a point can be shown usingCartesian Coordinates.

Here we see the point (12,5)

Line

In geometry a line:

• is straight (no curves),
• has no thickness, and
• extends in both directions without end (infinitely).

A line has no ends 


Line Segment

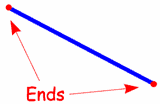
If it does have ends it is called a "Line Segment".

Ray

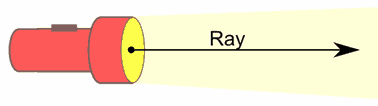
If it has just one end it is called a "Ray"

Degrees (Angles)

We can measure Angles in Degrees.

There are 360 degrees in one Full Rotation (one complete circle around).

(Angles can also be measured in Radians)

(Note: "Degrees" can also mean Temperature, but here we are talking about Angles)

The Degree Symbol: °

We use a little circle ° following the number to mean degrees.

For example 90° means 90 degrees

One Degree

This is how large 1 Degree is

The Full Circle

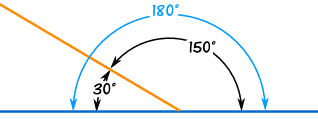
A Full Circle is 360°

Half a circle is 180°
(called a Straight Angle)

Quarter of a circle is 90°
(called a Right Angle)

Why 360 degrees? Probably because old calendars (such as the Persian Calendar) used 360 days for a year - when they watched the stars they saw them revolve around the North Star one degree per day. Also 360 can be divided evenly by 2, 3, 4, 5, 6, 8, 9, 10, 12, 15, 18, 20, 24, 30, 36, 40, 45, 60, 72, 90, 120 and 180, which makes a lot of basic geometry easier.

Measuring Degrees

We often measure degrees using a protractor:

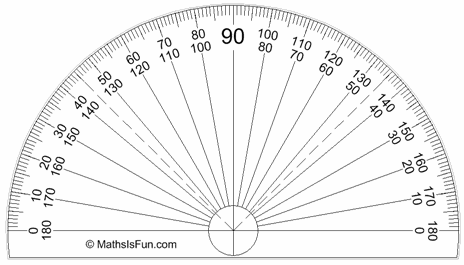
The normal protractor measures 0° to 180°

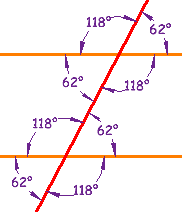
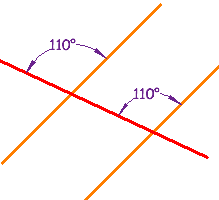
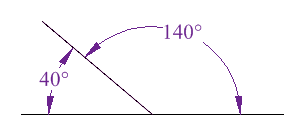
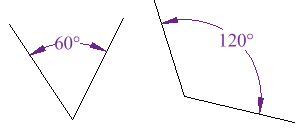
Supplementary Angles Two Angles are Supplementary when they add up to 180 degrees. These two angles (140° and 40°) are Supplementary Angles, because they add up to 180°: Notice that together they make a straight angle. But the angles don't have to be together. These two are supplementary because 60° + 120° = 180° Play With It ... (Drag the points) 55° + 125° = 180° Complementary Supplementary © 2015 MathsIsFun.com v 0.82 When the two angles add to 180°, we say they "Supplement" each other.  Supplement comes from Latin supplere, to complete or "supply" what is needed. 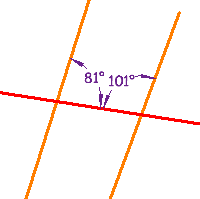Spelling: be careful, it is not "Supplimentary Angle" (with an "i") Complementary vs Supplementary A related idea is Complementary Angles, they add up to 90° How to remember which is which? Easy! Think: "C" of Complementary stands for "Corner"  (a Right Angle), and "S" of Supplementary stands for "Straight" (180 degrees is a straight line) You can also think "Supplement" (like a Vitamin Supplement) is something extra, so it is bigger.

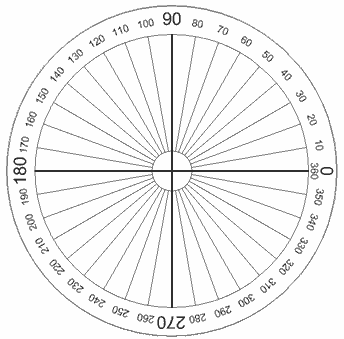
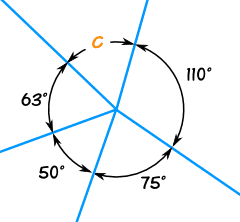
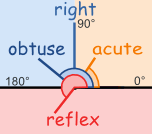
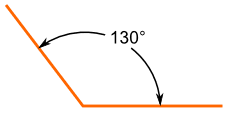
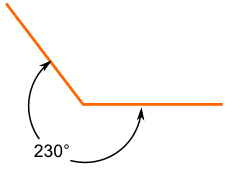
There are also full-circle protractors. But they are not as commonly used because they are a bit big and don't do anything special. Angles An angle measures the amount of turn Names of Angles As the Angle Increases, the Name Changes: Type of Angle Description Acute Angle an angle that is less than 90° Right Angle an angle that is 90° exactly Obtuse Angle an angle that is greater than 90° but less than 180° Straight Angle an angle that is 180° exactly Reflex Angle an angle that is greater than 180° Try It Yourself: © 2015 MathsIsFun.com v0.86 In One Diagram This diagram might make it easier to remember: Also: Acute, Obtuse and Reflex are in alphabetical order.   Also: the letter "A" has an acute angle. Be Careful What You Measure This is an Obtuse Angle And this is a Reflex Angle But the lines are the same ... so when naming the angles make sure that you know which angle is being asked for! 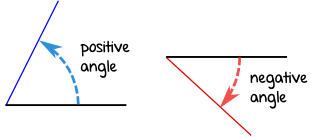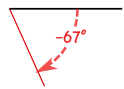Positive and Negative Angles When measuring from a line: 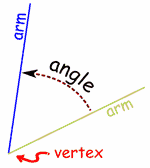a positive angle goes counterclockwise (opposite direction that clocks go) a negative angle goes clockwise Example: −67°   Parts of an Angle The corner point of an angle is called the vertex And the two straight sides are called arms The angle is the amount of turn between each arm. How to Label Angles There are two main ways to label angles: 1. give the angle a name, usually a lower-case letter like a or b, or sometimes a Greek letter like α (alpha) or θ (theta) 2. or by the three letters on the shape that define the angle, with the middle letter being where the angle actually is (its vertex). Example angle "a" is "BAC", and angle "θ" is "BCD" Parallel Lines, and Pairs of Angles Parallel Lines Lines are parallel if they are always the same distance apart (called "equidistant"), and will never meet. Just remember: Always the same distance apart and never touching. The red line is parallel to the blue line in both these cases: Example 1 Example 2 Parallel lines also point in the same direction. Parallel lines have so much in common. It's a shame they will never meet! Try it yourself: Pairs of Angles When parallel lines get crossed by another line (which is called aTransversal), you can see that many angles are the same, as in this example: These angles can be made into pairs of angles which have special names. Click on each name to see it highlighted: Choose One: © 2015 MathsIsFun.com v 0.81 Testing for Parallel Lines Some of those special pairs of angles can be used to test if lines really are parallel: If Any Pair Of ... Example: Corresponding Angles are equal, or a = e Alternate Interior Angles are equal, or c = f Alternate Exterior Angles are equal, or b = g Consecutive Interior Angles add up to 180° d + f = 180° ... then the lines are Parallel Angles On One Side of A Straight Line Angles on one side of a straight line will always add to 180 degrees. If a line is split into 2 and you know one angle you can always find the other one. 30° + 150° = 180° Example: If we know one angle is 45° what is angle "a" ? Angle a is 180° − 45° = 135° This method can be used for several angles on one side of a straight line. Example: What is angle "b" ? Angle b is 180° less the sum of the other angles. Sum of known angles = 45° + 39° + 24° Sum of known angles = 108° Angle b = 180° − 108° Angle b = 72° Angles Around a Point Angles around a point will always add up to 360 degrees.  The angles above all add to 360° 53° + 80° + 140° + 87° = 360° Because of this, we can find an unknown angle. Example: What is angle "c"? To find angle c we take the sum of the known angles and take that from 360° Sum of known angles = 110° + 75° + 50°  + 63° = 298° Angle c = 360° − 298° = 62° Examples These lines are parallel, because a pair ofCorresponding Angles are equal. These lines are not parallel, because a pair ofConsecutive Interior Anglesdo not add up to 180° (81° + 101° =182°) These lines are parallel, because a pair of Alternate Interior Angles are equal