Graphing Linear Inequalities
This is a graph of a linear inequality:
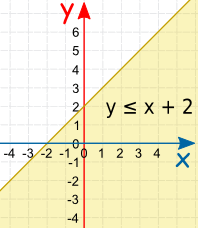
The inequality y ≤ x + 2
You can see the y = x + 2 line, and the shaded area is where y is less than or equal to x + 2
Linear Inequality
A Linear Inequality is like a Linear Equation (such as y = 2x+1) ...
... but it will have an Inequality like <, >, ≤, or ≥ instead of an =.
How to Graph a Linear Inequality
First, graph the "equals" line, then shade in the correct area.
There are three steps:
- Rearrange the equation so "y" is on the left and everything else on the right.
- Plot the "y=" line (make it a solid line for y≤ or y≥, and a dashed line for y<or y>)
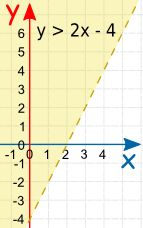
- Shade above the line for a "greater than" (y> or y≥)
or below the line for a "less than" (y< or y≤).
Let us try some examples:
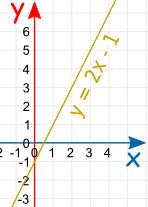
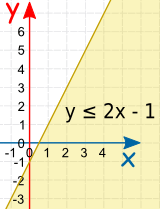
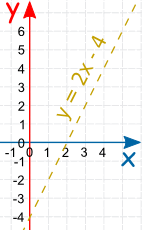
The dashed line shows that the inequality does not include the line y=2x-4.
Two Special Cases
You could also have a horizontal or vertical line:
 | 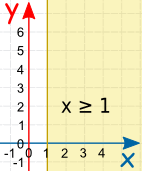 |
| This shows where y is less than 4 (from, but not including, the line y=4 on down) Notice that we have a dashed line to show that it does not include where y=4 | This one doesn't even have y in it! It has the line x=1, and is shaded for all values of x greater than (or equal to) 1 |

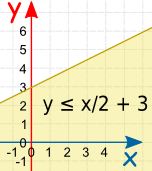
No comments:
Post a Comment